Jimmy Garnier
Chargé de Recherche CNRS, Université de Savoie
Research interests
According to theoretical and experimental ecologists, there is a need in quantitative models that are able to cope with the complexity of issues arising in life science. The great challenge of my research is to develop and analyze complex PDEs models for biological propagation and adaptation phenomena. My strong interactions with ecologist needed to provide relevant and meaningful models and feedback on applications as well as the development of new mathematical approaches required to perform the analyze, are two powerful sources of motivation for my research. As an applied mathematician, I publish articles in mathematical journals, applied mathematics or theoretical ecology journals or even in general scientific journals. Here are 4 selected articles showing the diversity of my scientific research with strong interactions with ecology.
Mathematics:
Nonlinear integral model in heterogeneous media
- V Calvez, J Garnier and F Patout. A quantitative genetics model with sexual mode of reproduction in the regime of small variance, J Ecole polytechnique --- Mathematiques, (2019).
Recent developments in evolution biology have proposed a new quantitative PDE model to understand the complex phenomenon of adaptation in sexual population. The mathematical challenge is to understand the convergence towards a stationary distribution observed in many experiments. Conversely to previous studies, we use perturbative analysis techniques combined with rigid contraction properties to construct our stationary solutions. Our alternative methodology extends previous results obtained with linear operators and it provides a general approach to understand spectral properties of nonlinear operators.
Accelerating phenomena in integro-diferrential models
- E Bouin, C Henderson,, J Garnier et F Patout. Thin front limit of an integro--differential Fisher--KPP equation with fat--tailed kernels, SIAM J. Math. Anal., (2017).
Accelerating propagation phenomenon has been reported experimentally and theoretically in various scientific field (ecology, physics, evolution) and many PDE models reproduce this behavior. However, this phenomenon is far from being well understood. We have developed a new general method, classical PDE technics (sub and super-solutions) with geometrical optics approach, to describe the accelerating solutions of these models.
Ecology:
Multi-species model: emergence and stability of mutualism
- M Martignoni, M M Hart, R Tyson and J Garnier, Diversity within mutualist guilds promotes coexistence and reduces the risk of invasion from an alien mutualist, Proc. R. Soc. B, (2020).
Green fertilizers based on Arbuscular Mycorrhizal Fungi (AMF) seems to be a relevant and ecological alternative to chemical fertilizers. But what is the impact of the inoculation of these industrial fertilizers on wild-type AMF communities? Although predator-prey systems receive a lot of attentions, mutualistic systems are badly understood. Here, we provides a theoretical framework to explore the stability and the spreading properties of this system. In particular, we show that diversity is a source of stability among this system.
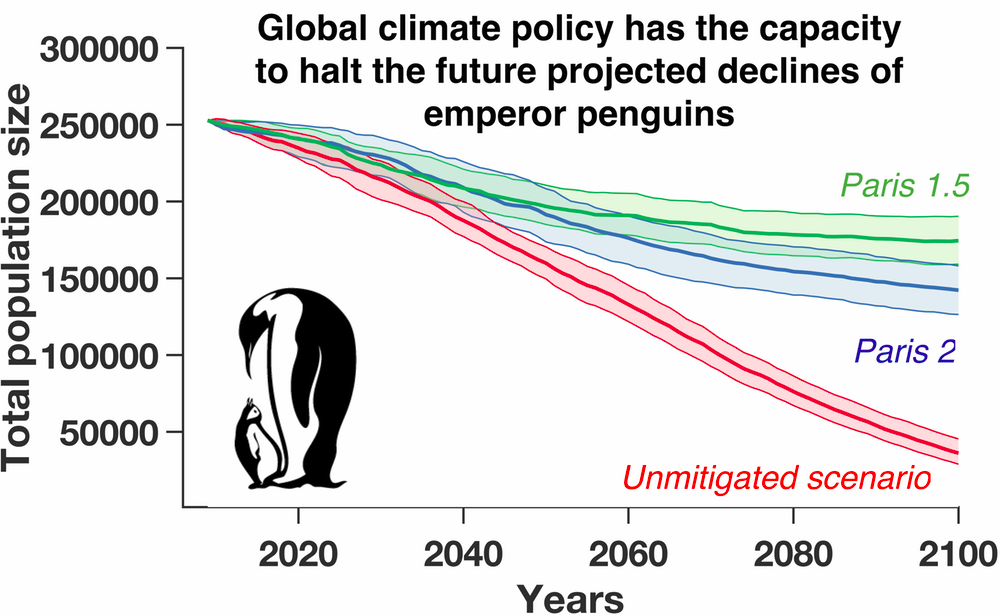
Demographic and genetic consequences of climate change
- S Jenouvrier, M Holland, D Iles, S Labrousse, L Landrum, J Garnier, H Caswell, H Weimerskirch, M La Rue, R Ji, C Barbraud . The Paris Agreement objectives will likely halt future
declines of emperor penguins, Global Change Biology, (2020);
Understanding the ecological consequences of climate changes are a major issue of our century. We focus on an emblematic species of Antarctica, the Emperor Penguin (Aptenodytes forsteri). By combining our mathematical model on this species dynamics and the most recent climate projections, we have shown that if climate change continues at its current rate, Emperor Penguins could virtually disappear by the year 2100 due to loss of Antarctic sea ice. However, a more aggressive global climate policy such as the Paris' agreement on climate, can halt the penguins’ march to extinction.
- S Jenouvrier, M Holland, D Iles, S Labrousse, L Landrum, J Garnier, H Caswell, H Weimerskirch, M La Rue, R Ji, C Barbraud . The Paris Agreement objectives will likely halt future
declines of emperor penguins, Global Change Biology, (2020);